Process dimensions of mathematics
Overview
Includes information to help learn and facilitate the learning and use of the process dimensions of mathematics.
Problem solving Tools to facilitate mathematical problem solving
- Problem solving Concepts, misconceptions, & outcomes by levels in the mathematical knowledge base
Information to facilitate literacy in problem solving & tools to achieve it
- Development of problem solving - page contents includes:
- Heuristic:
- Strategies for solving problems with sample problems
- Planning & teaching tools pedagogical and curriculum
- Teacher's role in problem solving
- Suggestions to help learners solve problems
- Assessment sample questions to assess learners' mathematical problem solving
- Characteristics of a good problem ideas to consider when writing or selecting problems
- Planning questions to prepare to facilitate problem solving & investigating suggestions to consider when writing or planning to use problems
- Problem Solving Goals and Outcomes to review for the inclusion in appropriate curricular documents and plans
- Problem solving vocabulary to introduce to learners
- Dispositions to review & select to focus on
- Learner's materials
- Learners problem solving guide or checklist to use to as a guide with suggestions to solve problems
- Learners solving checklist - with before, during, and after suggestions
- Learners Solving Attitude Inventory with yes - no questions
- See also communication dimension -
Activities to facilitate problem solving
Reasoning and proof
- Reasoning & proof Concepts, misconceptions, & outcomes by levels in the mathematical knowledge base
Information to facilitate literacy in reasoning & proof & tools to achieve it
- Reasoning and Proof Explanations and development of reasoning, proof, & logic - information on reasoning and proof with examples of different types of proof, teaching suggestions, & an extensive scoring guide
- Reasoning and proof big idea, concept, or generalization instructional planning map
- Development of reasoning from birth to maturity
- Reasoning, conservation, and cognitive development tasks & activities
- Application of proof by elimination & verification with a card trick
- Bucket problems reasoning, proof, conjecture, combinations
- Decision making, critical thinging, and change processes
- Structure for Analyzing and Presenting Arguments
- Logical Reasoning and Reasoning Errors
- Position analysis
- Issue analysis & sample issues analysed for democratic education for all
- Reasoning solutions for SUDOKU
- Imagining
- An Outline of Goals for a Critical Thinking Curriculum and Its Assessment
Activities to facilitate reasoning & proof
Representation
Representation Concepts, misconceptions, & outcomes by levels in the mathematical knowledge base
Information to facilitate representation & tools to achieve it
- Examples of mathematical representations with language as nouns and verbs.
- Function exploration - includes definitions with examples, diagrams, & representations including a function machine. Also activity with work sheet and charts to explore a function with a variable factor (3) & constant factor (27) & discover casting out nines
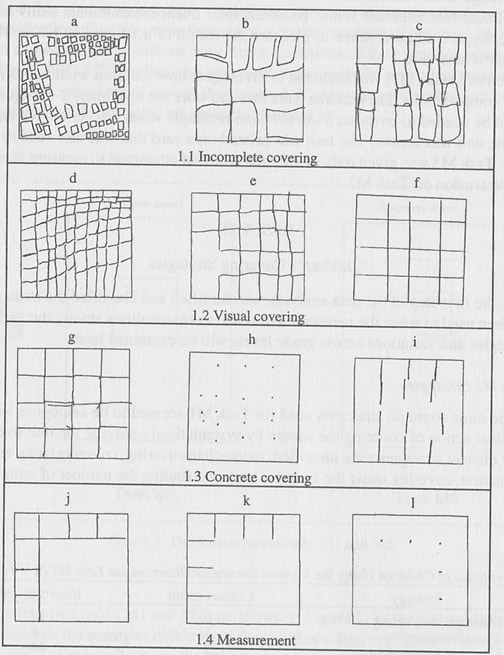
Area representations
Lynne Outhred and Michael Mitchelmore asked learners to illustrate the area of a rectangular shape. They found learners demonstrated four different strategies: incomplete covering, visual covering, concrete covering, and measurement. The drawings below show samples from each.
Representation of relative position of objects
Illustration of Internal Representation

Actual object, real world, or external representation
Representation of external objects with points on an other external object

What does this have to do with mathematical representations?
Activities to facilitate representation
Communication
Communication Concepts, misconceptions, & outcomes by levels in the mathematical knowledge base
Information to facilitate literacy in communitation & tools to achieve it
- Different ways to communicate problems with sample problems
- Ways to communicate mathematical representations as objects, properties, actions, & operations
- Graphing with line graphs as communication
Activities to facilitate reasoning & proof
Connections & perspective
Connections & perspective Concepts, misconceptions, & outcomes by levels in the mathematical knowledge base
Information to facilitate literacy in connections & perspective & tools to achieve it
- Mathematical ideas build upon each other.
- Mathematical ideas are connected to other mathematical ideas.
- Mathematical ideas are connected to the world.
Activities to facilitate reasoning & proof
Contents:
Other math knowlege base &
activity pages
Content dimensions
Common sense & knowledge
Counting in Winnebago
- WIn
- NOnBA
- THABTHIn
- DUBA
- SATOn
- SHAPE
- PETHOnBA
- PETHABTHIn
- SHOnKA
- GTHEBOn
Interesting book

The Power of Logical Thinking: Easy Lessons in the Art of Reasoning... and Hard Facts About Its Absence in Our Lives, Marilyn Vos Savant (1996) ISBN 0-312-13985-3 Saint Martin's Press: New York.

