Development of whole number sense

Introduction

Overview

This article includes concepts and processes necessary to understand whole number values and their relationships. Suggestions on how children develop them, use them, and ideas on how to understand these learners to make better pedagogical decisions to facilitate the conceptualization of the ideas necessary for number literacy.

Information starts with a counting overview and follows with prenumber sense and whole number sense.

Prenumber sense focuses on concepts and algorithms necessary for basic cardinality; and whole number sense continues on toward the development of relationships of the values of whole numbers and information needed to develop place value, other number sense and basic operations.

Counting

When people think of counting they usually think of two big ideas:

  1. To repeat numbers in numerical order.
  2. Count the number of objects in a group (set). Cardinality.
  3. Number sense based on the continuous systematic order of whole numbers.

People often wrongly assume: when a person can count, they know and can use number value relationships between the numbers.

However, that is not true. There is an enormous difference in what people understand as necessary to learn to count and make sense of whole numbers.

The following list identifies concepts or algorithms associated with counting and making sense of whole numbers and their relationships:

This article begins to explain these complexities and how they can be organized, applied, and connected.

First, let's explain some of the organization. ... :

  • Concepts are grouped into two big levels: Prenumber sense ages 0 - 6 years and whole number sense ages beyond 6 years to fourth grade or middle school.
  • The list of concepts and algorithms identified above are necessary to count and develop a very good sense of whole number values and their relationships.
  • The learning of these concepts or algorithms sometimes depend on knowing ideas listed before another concept. However, students can work simultaneously on concepts in the list and develop a certain level of understanding, that can be a naive understanding, but important in knowing for connecting ideas as is necessary to eventually use one and then another to mathematize. For example students learn counting words a few at a time. Counting to ten at a younger age than to 20, and even later to 100, and much later to six digit numbers. At the same time they are learning these, they are capable of conceptualizing other concepts in the list. However, there are some which require much experience before being able to successfully achieve them.

Additionally

  • There are limitations in showing the importance of connections between and among these concepts by isolating and focusing on them individually. However, it is essential to understand each to orchestrate the connections between them to achieve mathematical literacy.
  • Learning to count takes time and is initially aided and hampered by our visual spatial skills. One example: conservation skills.
  • Counting is motivated by questions students ask such as: how many? are there more? less? or are they the same?
  • When students learn to count, they want to use it to solve all problems. This is detrimental for learning and using mathematics effectively, efficiently, and with understanding. Once students memorize number words sequentially, they should stop counting and we should help them move toward a greater understanding of the concepts included in whole number sense beyond prenumber sense. See activities for number sense and number value and how values related to different operations.

 

Pre Number Sense - Development (age 0 - six)

Classification

Classification or categorizing is integral to knowing and using numbers. Different ways to categorize and classify must first be applied to understand and identify the objects or what group of objects are to be counted, or described numerically, with a number value (cardinality or other numerical representation).

  • Children classify when they play: as they sort, organize, group and regroup objects. First by one general (dog, cat) property (or attribute) and later with specific (boxer, collie) properties.
  • Children use classification when they select objects to duplicate a pattern.
  • As mentioned above, children are first able to group by one property (big v. small or circle v. square). When asked to classify objects using multiple properties or attributes, (big square, small square, big circle, small circle), they find it difficult and can be assisted when examples are modeled with the use of Venn diagrams. With many opportunities they will eventually learn to classify groups (sets) of objects by multiple attributes (properties) and represent them with Venn diagrams.
  • After children classify, they may question: How many objects are in the groups they create? This leads to counting and eventually different ways to represent number value with number sense.
  • Additional information:

Counting words

Whole number counting words (one, two, three, ...) must be memorized. Memorized in order and associated with that order and eventually a value (cardinality). When student memorize counting words they usually learn them as connected words.

Counting as connected words

Sequences of number words are memorized: onetwothreefourfivesixseven Where the words are not differentiated and very few values are associated.

With an association objects to numerals, or number words, children differentiate the sequence of words into separate words: one two three four five six seven. Connecting an oral word to an object being counted, later to its numeral, and later to its written word. With lower values (1, 2, 3, ...) understood first and better than higher value numbers.

Therefore, counting words are first learned as connected words by rote. Allowing students to respond only with a counting sequence entirely from memory, without a connection to the value of numbers (cardinality) in the sequence for most numbers with values associated from smaller numbers to larger numbers as learners attempt a one-to-one correspondence of numbers to objects. ...

Synchrony

Synchrony is when learners attempt to connect one word to one object, but haven't conceptualized a perfect one-to-one correspondence yet. They do not systematically match number words to objects so some objects are counted more than once, or missed. Additionally, sometimes the objects being counted will out number the sequence of counting words they know. When this happens they will sometimes repeat their largest number, or stop when they get to it, or continue to recount objects until they get to it if there are too few objects. This larger number, for some, seems to represent their idea of a large group of objects, more than the cardinality of the group.

Thus, synchrony is the attempt to use one word for every object (1 - 1 tagging). It is developed before one-to-one correspondence. Students connect one word to one object, but not a perfect one-to-one correspondence yet. They seem not to know each object should be counted once and only once, or they have not systematized an algorithm to order objects so objects are counted once and none missed.

Young children will count objects without an accurate one-to-one correspondence, number conservation, systematic procedure. that includes these ideas:

[Counting overview]

One-to-one correspondence

One-to-one correspondence is when one numeral is related to one object being counted. Students pair words with objects. Students usually start by pointing to each object and moving each object as they count. Later point without touching.

One, two, three, four, five, six, seven

x........x......x......x......x....x......x

One-to-one correspondence is constructed before conservation of numbers.

Suggestions:

Once students know how to count they should be encouraged to develop more efficient strategies (subitizing and construction) to determine quantities (cardinality).

Activities

Counting systematically

With experience students will count objects in an organized manner to help them remember with what object to start counting, a path of objects to count each and every object, and with what object to stop the counting. Additionally they will understand the value will not change if objects are moved (conservation) , there by allowing them to put objects into pairs, groups of five, or tens to assist counting and accuracy.

Systematic counting includes:

  • An object ought to be selected to start the counting (starting line).
  • An object ought to be selected to stop the counting (finish line).
  • A path ought to be selected from the beginning object to the finishing object so each object can be included.
  • The path for counting should be such that each object is counted once and only once (one-to-one correspondence).
  • Objects can be rearranged to facilitate counting (conservation).
  • About the total value (cardinality).

Suggestions:

When students learn to count, they want to use it to solve all problems. This is detrimental for learning and using mathematics effectively, efficiently, and with understanding. Once students memorize number words sequentially, they should stop counting and we should help them move toward a greater understanding of number sense and number value and how values related to different operations.

[Counting overview]

Subitizing

Subitizing is being able to identify the value of a group of objects by looking at it without actually counting the objects. Children can do this at a very young age and seem to be able to understand one, two, and three as a perceptual magnitude not cardinality. Being able to perceive two or three as a whole without doing mathematical thinking can be done by birds and some other animals. Therefore, young children can label small groups, as two, three, or four, accurately by subitizing, but no cardinality maybe recognized for the group. However, as children mature and gain experience it can also be a confident judgment of cardinality for a small number of objects.

Subitize, comes from the Latin subitus meaning sudden, which represents the sudden dawning of that's three or four. It was suggested in 1949 by E. L. Kaufman, M. W. Reese, T. W. Volkmann, and J. Volkmann.

It is possible for children to identify two fingers as two fingers. Or say they have five fingers. Or that you are holding up three fingers, without knowing the value of the the numbers. Particularly for five and above. They can recognize the value of a group through perceptual recognition of visual patterns of the objects' relative positions (subitizing) or through procedural counting without understanding the group of object's cardinality.

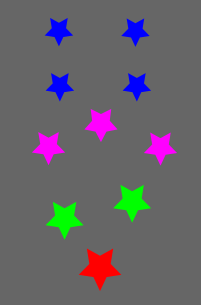
star image

Benefits, people can learn to subitize and develop skill with practice. The benefit of drilling students with subitization exercises, rather than counting or basic fact operations, will increase the student's ability in number sense. Relationships of number quantity, cardinality, conservation of numbers, operations, and proportion.

Also being able to recognize and apply it in different instances is a first step toward being able to use mathematics in a flexible and creative way. With practice students will be able to quickly subitize values of subgroups within a larger group and mentally join the subgroups to find the total value of the larger group.

Suggestions:

Additional ideas on subitizing

Conservation of numbers

Conservation of numbers must be constructed before cardinality. Children conserve numbers when they know that the value (cardinality) of a group of five is five no matter where the five objects are placed. No matter if the five horses are gathered by the fence or are in the barn or if they are spread out across a whole 10 acre field. Or the value of a group of five objects is the same as the value of a different group of five objects. Even if the size of objects is different. Say five elephants or five mice. See also:

Cardinality or Number value

Counting objects results in a cardinal result (numbered value). When young learners have the idea counting results in a final number, they will repeat or emphasize the last word to differentiate between a counting number and the total value of the group of objects, they identify the value (cardinality) with great assurance.

Will say: "one two three four five six seven, seven."
Will represent seven --->>> x x x x x x x

Determining cardinality by counting requires: knowing how to classify or create a group, knowing number words in sequence (counting sequence), one-to-one correspondence, conservation of number, systematic organization of objects, and numbers have values when connected to real objects.

Early learners, who can count systematically can count and determine the cardinality of a group of objects and not have a good sense of its whole number value or its relationship to other whole numbers. For example: Learners may count two quantities with one quantity being one more or less than the other and know the cardinalities (values), but not know that the second quantity is one more or one less than the first.

Cardinality can be determined in ways other than one-to-one counting. subitizing, pattern recognition (recognition of objects' relative positions relative to a cardinality related to the positioning) or by construction and deconstruction of subsets (recognize five dots on a die as 3 + 2). Amounts such as one, two, and three can be recognized and combined in a variety of ways for quick recognition of larger groups without counting. Similarly five can be recognized or constructed as an anchor and combined with other groupings such as a group of five and a group of two as seven. Concepts for a well developed whole number sense (number relationships, hierarchical inclusion, and operations) will give learners these and other ways to determine cardinality without counting, which is detrimental in developing a higher degree of number sense.

Resources: cardinality rubric

Counting down or back

Counting down requires memorization of counting numbers and the ability to remember them in the reverse order in which they are usually learned.

As learners develop more number sense, they will solve problems by knowing the order of numbers and the differences between them.

Zero and numbers beyond ten

  • Value of zero as absence of something or a starting point.
  • Zero is equivalent to n - n

Activities

  • Use an empty plate to represent zero objects on the plate.
  • Ask questions such as how many elephants in the room, zero.
  • Use bags of objects to sequence numbers starting with an empty bag for zero. Later combine objects in one bag to zero objects in another bag. Suggest several problems with zero as an addend and include both kinds of problems 3 + 0 = 3 and 0 + 3 = 3. Have others make and share problems.

Use of zero as a place saver is problematic for students not only as a place saver, but because it has two functions: place saver, and value of nothing. Similarly it becomes problematic for students to realize that as other numerals are moved into different positions they can simultaneously represent values other than the counting value they originally learned for them.

It is easier for children to understand the values of the numerals 11, 12, 13,... than the value of the numeral 10. It is easier to understand the values of 14, 15, 16,... than 11, and 12. In some languages these values are said as ten and one, ten and two, ten and three, and so on ...

Conclusion

While there may not always be an exact demarcation between PreNumber sense and number sense the understanding of cardinality, which includes: knowing how to classify or create a group, number sequence, counting sequence, counting systematically, synchrony, one-to-one correspondence, conservation of number, systematic organization of objects, and numbers have values when connected to real objects, is being used here. For information See PreNumber sense (0 - age 6)

Number sense includes these ideas and extends them by including the idea that numbers can be represented in multiple ways and have relationships to other numbers.

 

 

Whole Number Sense - Development for age 6 - 8+
Concepts, sample activities, & assessment ideas

Number notation

  • Symbols can be used to represent numbers (number words (one...) and numerals (1, 2, ...)
  • Symbols can be used to represent relationships (< > =) equal, not equal, greater than, less than or number or sets.
  • Symbols can be used to represent operations (+ - * /) with numbers.
  • Simple number sentences include numbers, relationships and operations.
  • Equations are number sentences, which claim to state equal or unequal relationships (equality or inequality).

Number relationships

  • Numbers are related to other numbers through a variety of relationships. These relationships of numbers can be compared as less (<), greater (>), equal (=), and unequal (≠).
  • Equivalency is a comparison of two groups with the same value (cardinality).
  • Greater than and less than is understood when:
    1. the value of numbers is known (cardinality),
    2. conservation of numbers is possible,
    3. ordinality is understood,
    4. and can be represented symbolically if the symbols and their meaning are known and understood.

Simple one to one relationships

  • Numbers can be compared in value as greater than, less than, or equal to another.
  • A relationship of one more exists for all numbers.
  • One less exists for all whole numbers except zero.
  • Two more exists for all numbers, three more, ...
  • Two less exists for all whole numbers greater than two, three less, ...

Addends can be independent cardinal values and part of the sum of the two numbers.

 

  • one two three four = four ........one two three = three
  • x.. . ... x. .... x....... x................ ....... o.... o..... o
  • one two three four five six seven = seven
  • x ......x..... x....... x.... o... o..... o
  • They will count out four (cardinality for four). Count out three more (cardinality for three). Put them together and count the total (seven). They will recognize that both sequences make up the sequence of seven.

Counting on

The sequence words can be cardinal values and are recognized as embedded in the total.

0 0 0 0 ............ x ... x ..... x

....... four ....... five, six, seven

Recognize Smaller sequences are included in larger sequences.

Students will recognize that four plus three are both included in seven.

 

Ordinality

Objects can be ordered by number values (numerically) according to their relative position.

 

Relationships of one to many, many to one, and many to many

  • Numbers can be represented in different forms. (four, 4, 2+2, 3+1, 4+0, 3+1=4+0,...)
  • Smaller sequences can be included in larger sequences (inclusion) (1,2,3; is also in 1,2,3,4 and so on). (number sequence and counting).
  • Sequences of counting can be joined to make longer sequences. Counting from one to four can be joined with counting from one to three and it is the same as counting from one to seven. Or counting from 1 to 3 and 1 to 4 is the same as from 1 to 7.
  • Addends have independent cardinal values (5 and 3) and are part of the sum (8) of the two numbers (5 + 3 = 8).
  • Simple patterns can be recognized in more complicated patterns (inclusion) (squares in squares, 1=0+1,1+0; 2=0+2,1+1,2+0; 3=0+3,1+2,2+1,3+0).
  • Parts can be compared to other parts and wholes (7 = 3 + 4 and 3 + 4 = 5 + 2).
  • Equivalency is a comparison of one number or more numbers combined with an operation to another number or two or more numbers combined by an operation
    (5 = 2 + 3; 4 + 1 = 5; 3 + 2 = 0 + 5).

Hierarchical inclusion

Hierarchical inclusion of a counting sequence relates to number value and addition

  • A part can be compared to a whole. Take a whole of 5, it can be composed of 3 + 2 = 5; therefore, in this case, a part (3) is less than the whole (5), 3 < 5; or 3 is two less than 5.
  • Parts can be compared to wholes, part + part compared to whole (2 + 3 compared to 5 )
  • Hierarchical inclusion for addition is knowing that each number has all combinations of addends for numbers before it:
    5 = 0+5, 1+4, 2+3, 3+2, 4+1, 5+0.
  • Addends can be independent cardinal values and part of the sum of the two numbers.

one, two, three, four = four .... & .... one, two, three = three;
x........x........x.......x................ & ..........o......o.......o; is the same as seven
one two three four five six seven = seven;
x.......x......x.......x......o....o.....o .... = ...7...

Hierarchical inclusion assessment

Totally embedded cardinality of numbers

Know each number can be created as combinations of addends for all numbers below.

5 = 0+5, 1+4, 2+3, 3+2, 4+1, 5+0

Hierarchical inclusion of a counting sequence (cardinality)

  • The idea that all numbers preceding a number can be or are systematically included in the value of another selected number.
  • The idea that yellow cube isn't just the third object in a group of four cubes, but also represents all three cubes in the set of four cubes.
  • The numbers 1, 2, 3 are also included in 1, 2, 3, 4. Or every number below any selected number is included in a counting sequence when counting to that number.
  • Every number contains the sequence of all the numbers that are smaller than the largest number in any sequence. A totally embedded sequence for cardinality of all numbers.
  • Know that
    • 1
    • 1 2
    • 1 2 3
    • 1 2 3 4
  • Know what numbers come before and after a number in a sequence (one more and one less). They must conserve and know cardinality.
  • Know that ordinality is contained within cardinality and there is a total embedded reversibility of sequences for cardinality and ordinality of numbers.

At a beginning level of hierarchical inclusion a student could be asked to count some objects (seven) and put them in a container. Then a person takes some out (three), covers the container with the remaining, and asks how many are remaining. If they can answer correctly (four), then they have constructed hierarchical inclusion.

Counting on

  • The sequence words can be cardinal values and are recognized as embedded in the total.
  • To count on students must know cardinality and hierarchical inclusion of the counting sequence.

Instead of counting to four for the following group of o and x's.

...........0 0 0 0........................... x ...x..... x
They say four and count ............ five, six, seven

Count back

Imagine a set of 8 objects with the eighth, seventh, and sixth object being removed (8 - 3 = 5), leaving 5. Or a group of eight objects with 8, 7, 6 being removed to leave 5.

The sum of three addends in a sequence are equal to the sum of two addends in an equal sequence

7 + 6 = 12 + 1 = 13

because 7 = 6 + 1, and 6 + 6 = 12, and 6 + 6 + 1 = 13, therefore 6 + 7 = 13

Number value and operations

It is helpful to compare numbers to groups of five and ten (five as an anchor and significance of ten in place value) .

A missing part can be found if the total and left over amounts are known

The sum of three addends in a sequence are equal to the sum of two addends in an equal sequence: 6 + 6 + 1 = 7 + 6. Used for solving the problem of 7 + 6 by thinking of it as 12 + 1 = 13; because the 7 can be decomposed into 6 + 1, and 6 + 6 can be thought of as 12, then add the one. Simplified as 6 + 6 + 1 = 13, therefore 6 + 7 = 13

Resources

Count back

8 - 3 = 5

Imagine a set of 8 objects with the eighth, seventh, and sixth object being removed, leaving 5.

8, 7, 6 removed and 5 left

Nominal numbers

Nominal numbers are used for names. She is number four on the team.

Infinite

  • Infinite has no bounds or limits
  • There can be infinite number systems (Roman, Mayan, Egyptian, and Greek

Next see development of Place value concepts

Addition and subtraction checklist

 

Home - Mathematics

Top

Dr. Robert Sweetland's notes
[ Home homeofbob.com & schoolofbob.com ]